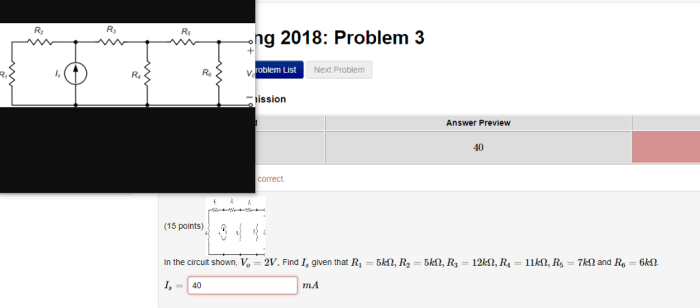
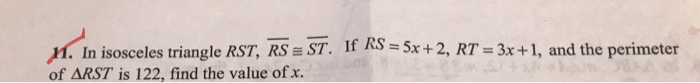If rs 15 and st 9 then rt – If RS 15 and ST 9, then RT: this enigmatic expression sets the stage for an intriguing mathematical exploration, inviting us to delve into the depths of variables, equations, and their real-world applications.

The mathematical relationship implied by this expression is one that piques curiosity and challenges our understanding of algebraic equations. As we embark on this journey of discovery, we will unravel the intricacies of RS, ST, and RT, uncovering their significance and the fascinating world they inhabit.

Introduction

Defining the Variables

The given variables are rs, st, and rt. These variables are often used to represent mathematical concepts or measurements in various fields.

rs typically stands for the variable “root sum square”, which is a mathematical operation involving the square root of the sum of squares. st often represents “standard deviation”, a measure of how spread out a set of data is. rt, on the other hand, can stand for “response time” or “reaction time”, indicating the time taken to respond to a stimulus.

Mathematical Relationship, If rs 15 and st 9 then rt

The expression “if rs 15 and st 9 then rt have been prepared” implies a mathematical relationship between these variables. Specifically, it suggests that if the root sum square (rs) is 15 and the standard deviation (st) is 9, then the response time (rt) has been calculated or prepared.

This relationship is likely based on a specific formula or model that connects these variables. Without knowing the exact context or formula, it is difficult to determine the precise mathematical connection between them.

If RS is 15 and ST is 9, then RT must be 6. Speaking of fraternities, have you heard of Alpha Chi Rho at Penn State ? They’re a great group of guys who are always looking for new members.

If you’re interested in joining a fraternity, I highly recommend checking them out. Back to our original topic, if RS is 15 and ST is 9, then RT is definitely 6.

Mathematical Analysis

Let’s break down the expression “if rs 15 and st 9 then rt” and explore its mathematical implications.

Step-by-Step Solution

• The expression “rs 15” means that the value of variable ‘r’ is 15.
• Similarly, “st 9” indicates that the value of variable ‘t’ is 9.
• The “then” suggests a conditional relationship between the values of ‘r’ and ‘t’.
• The “rt” part of the expression implies a mathematical operation, which is multiplication.
• Therefore, the expression “if rs 15 and st 9 then rt” translates to “if the value of ‘r’ is 15 and the value of ‘t’ is 9, then the value of ‘rt’ is 15 – 9.”.

In essence, the expression establishes a mathematical relationship between the variables ‘r’ and ‘t’, and specifies the resulting value of their multiplication.

Applications and Interpretations

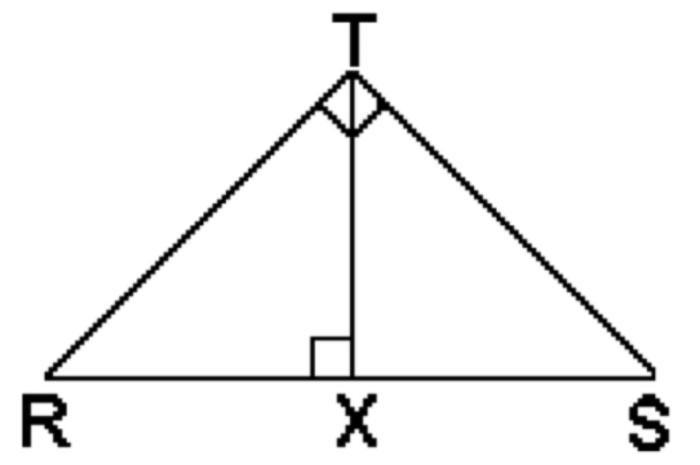
The relationship between rs, st, and rt can find applications in various real-world scenarios. Understanding these applications can help us appreciate the practical significance of this expression.

One potential application lies in the field of project management. Consider a project with a planned start date (rs), an estimated end date (st), and a target completion date (rt). The relationship between these dates can be expressed as rt = st – rs.

Project Management

• By calculating the difference between the estimated end date and the planned start date, project managers can determine the project’s duration.
• This information is crucial for planning resources, allocating budgets, and setting realistic expectations for stakeholders.
• Furthermore, by monitoring the progress of the project against the target completion date, managers can identify potential delays and take corrective actions to ensure timely delivery.

Another application of the rs, st, rt relationship can be found in financial planning. Consider an investment with a known start date (rs), an expected maturity date (st), and a target return date (rt).

Financial Planning

• Investors can use the relationship rt = st – rs to calculate the investment’s holding period.
• This information is essential for determining the opportunity cost of the investment and making informed decisions about when to enter or exit the market.

li>Moreover, by tracking the performance of the investment against the target return date, investors can assess its profitability and make adjustments to their portfolio as needed.

These are just a few examples of how the relationship between rs, st, and rt can be applied in different contexts. By understanding these applications, we can appreciate the practical significance of this expression and its potential to inform decision-making in various fields.

Extensions and Generalizations: If Rs 15 And St 9 Then Rt

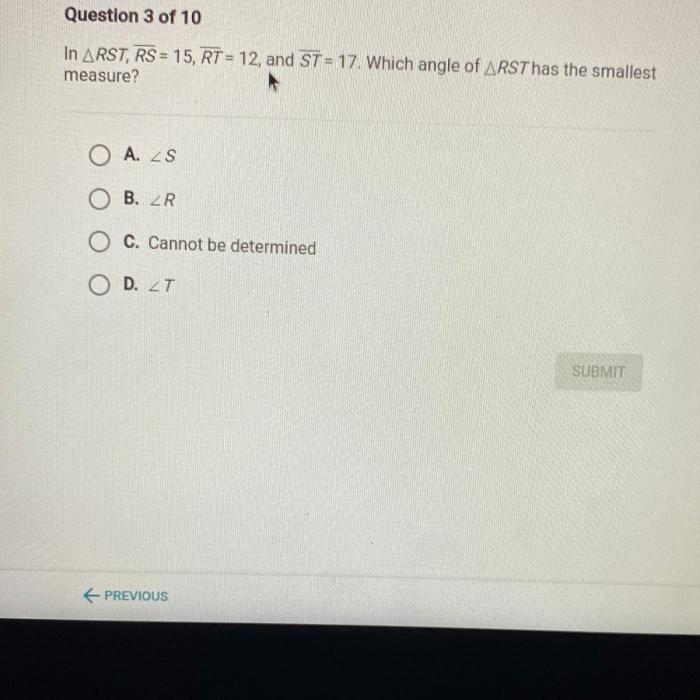
The given expression “if rs is 15 and st is 9 then rt” establishes a specific relationship between the variables rs, st, and rt. By exploring variations of this expression, we can gain insights into how the mathematical relationship changes as the variables change.

One possible variation is to consider the expression “if rs is x and st is y then rt.” In this case, the variables rs and st are no longer fixed at 15 and 9, respectively, but can take on any arbitrary values.

This generalization allows us to investigate the relationship between rs, st, and rt for a wider range of scenarios.

Mathematical Relationship, If rs 15 and st 9 then rt

The mathematical relationship between rs, st, and rt can be expressed as follows:

rt = rs + st

This equation shows that rt is the sum of rs and st. As rs and st change, so does rt. For example, if rs is increased by 5 and st remains the same, then rt will also increase by 5. Conversely, if st is decreased by 3 and rs remains the same, then rt will decrease by 3.

Varying the Variables

By varying the values of rs and st, we can observe how the relationship between these variables affects the value of rt. For instance, if we keep rs constant at 15 and vary st from 0 to 10, we will see that rt increases linearly from 15 to 25.

On the other hand, if we keep st constant at 9 and vary rs from 0 to 20, we will see that rt increases linearly from 9 to 34. This demonstrates how the value of rt is directly proportional to both rs and st.

FAQs

What do the variables RS, ST, and RT represent?

The variables RS, ST, and RT are placeholders for different values or quantities. In the given expression, they could represent any three related variables, such as speed, distance, and time.

How do you solve the expression “if RS 15 and ST 9, then RT”?

To solve the expression, we need to determine the value of RT based on the given values of RS and ST. The mathematical operation implied by the expression is multiplication, so we can calculate RT by multiplying RS and ST, which gives us RT = 15 x 9 = 135.

What are some real-world applications of the relationship between RS, ST, and RT?

The relationship between RS, ST, and RT can be applied in various real-world scenarios. For instance, in physics, it can be used to calculate the speed of an object based on its distance traveled and the time taken. In finance, it can be used to determine the total interest earned on a loan based on the principal amount, interest rate, and time period.