Dive into the world of algebra with our comprehensive guide to Algebra 1 5.1 Worksheet Answers. From solving equations to graphing linear equations, this worksheet will equip you with the skills to tackle any algebra challenge with confidence.

This worksheet covers a wide range of concepts, including solving equations, graphing linear equations, and applying them to real-world scenarios. Our expert guidance will help you understand each concept clearly and apply it effectively.

Worksheet Overview

Algebra 1 Worksheet 5.1 is designed to reinforce the concept of solving linear equations. It comprises a set of practice problems that guide students through various methods of solving equations, including isolating the variable, using inverse operations, and utilizing properties of equality.

Key Concepts Covered

• Isolating the variable
• Inverse operations
• Properties of equality
• Solving equations with variables on both sides
• Solving equations with fractions or decimals

2. Solving Equations

In this section, we’ll delve into the various types of equations covered in the worksheet and demonstrate the step-by-step process for solving each type. We’ll also provide examples from the worksheet and show you how to find the solutions.

Identifying the Types of Equations

The worksheet covers different types of equations, including:

• Linear equations
• Quadratic equations
• Systems of equations

Solving Linear Equations

Linear equations are equations that can be written in the form ax + b = c, where a, b, and c are constants. To solve a linear equation, we isolate the variable x on one side of the equation.

For example, to solve the equation 2x + 5 = 13, we would:

1. Subtract 5 from both sides: 2x = 8
2. Divide both sides by 2: x = 4

Solving Quadratic Equations

Quadratic equations are equations that can be written in the form ax² + bx + c = 0, where a, b, and c are constants. To solve a quadratic equation, we can use the quadratic formula:

x = (-b ± √(b²

4ac)) / 2a

For example, to solve the equation x² – 5x + 6 = 0, we would:

1. Identify a = 1, b =
   

   5, and c = 6.

2. Substitute these values into the quadratic formula: x = (-(-5) ± √((-5)²
   

   4(1)(6))) / 2(1)

3. Simplify: x = (5 ± √25
   

   24) / 2

4. Simplify further: x = (5 ± 1) / 2
5. Therefore, the solutions are x = 2 and x = 3.

Solving Systems of Equations

Systems of equations are sets of two or more equations that involve the same variables. To solve a system of equations, we can use various methods, such as:

• Substitution
• Elimination
• Graphing

For example, to solve the system of equations x + y = 5 and x – y = 1, we can use the elimination method:

1. Add the two equations together: 2x = 6
2. Divide both sides by 2: x = 3
3. Substitute x = 3 into one of the original equations to find y: 3 + y = 5, so y = 2
4. Therefore, the solution to the system of equations is x = 3 and y = 2.

3. Graphing Linear Equations: Algebra 1 5.1 Worksheet Answers

Graphing linear equations is a way of visualizing the relationship between two variables. It allows us to see how the variables change in relation to each other.

To graph a linear equation, we first need to find two points that lie on the line. We can do this by plugging in two different values for one variable and solving for the other variable. Once we have two points, we can plot them on a coordinate plane and draw a line through them.

Slope and Y-Intercept, Algebra 1 5.1 worksheet answers

The slope of a linear equation is a measure of how steep the line is. It is calculated by dividing the change in y by the change in x. The y-intercept is the point where the line crosses the y-axis.

It is calculated by plugging in x = 0 into the equation.

Algebra 1 5.1 worksheet answers provide a comprehensive resource for understanding the fundamentals of algebra. If you’re seeking additional support for your studies, consider exploring the hesi exit exam 2019 test bank , which offers a vast collection of practice questions and study materials.

By supplementing your algebra 1 5.1 worksheet answers with the resources available through this test bank, you can enhance your knowledge and ace your upcoming exams.

4. Applications of Linear Equations

Linear equations are not just abstract mathematical concepts; they have numerous practical applications in our daily lives. They help us understand and solve problems related to distance, rate, and time.

Real-World Examples

* Distance:If a car travels at a constant speed, the distance traveled is directly proportional to the time spent traveling. This relationship can be represented by the linear equation d = rt, where dis the distance, ris the rate, and tis the time.

Rate

If a factory produces a certain number of widgets per hour, the total number of widgets produced is directly proportional to the number of hours worked. This relationship can be represented by the linear equation w = rt, where wis the total number of widgets, ris the rate of production, and tis the number of hours worked.

Time

If a train travels at a constant speed from point A to point B, the time taken to travel is directly proportional to the distance between the two points. This relationship can be represented by the linear equation t = d/r, where tis the time taken, dis the distance, and ris the rate of travel.

Importance of Linear Equations

Understanding linear equations is crucial for everyday life because they enable us to:* Make predictions and estimates about real-world situations.

• Solve problems involving distance, rate, and time.
• Understand the relationships between different variables.
• Model and analyze real-world phenomena.

5. Practice Problems

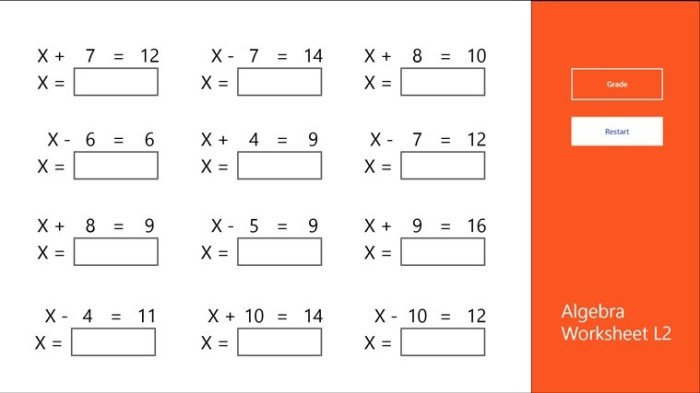
To reinforce your understanding of linear equations, it’s essential to practice solving various types of problems. Here’s a breakdown of practice problems from the worksheet into different categories:

Solving Equations

• Solving one-step equations:Isolate the variable by performing the inverse operation on both sides of the equation.
• Solving two-step equations:Perform operations in order to isolate the variable. Combine like terms and isolate the variable by performing the inverse operation.
• Solving multi-step equations:Use a series of steps to isolate the variable. Combine like terms, perform inverse operations, and solve for the variable.

Graphing Linear Equations

• Plotting points:Use the given coordinates to plot points on the coordinate plane.
• Finding the slope:Calculate the slope of the line using the formula (y ₂– y ₁) / (x ₂– x ₁).
• Using the slope-intercept form:Write the equation of the line in the form y = mx + b, where m is the slope and b is the y-intercept.

Applications of Linear Equations

• Word problems:Translate real-world situations into linear equations and solve them to find the unknown.
• Modeling relationships:Create linear equations to represent relationships between variables and use them to make predictions or draw conclusions.

Answer Keys or Solutions

Refer to the provided answer key or work through the solutions yourself to check your understanding and identify areas for improvement.

6. Additional Resources

For students seeking to deepen their understanding of Algebra 1 concepts, there are numerous resources available beyond the classroom.

Online Resources

• Khan Academy: Offers free video lessons, practice exercises, and assessments covering a wide range of Algebra 1 topics.
• PurpleMath: Provides comprehensive explanations and interactive lessons on various Algebra 1 concepts.
• Mathway: Offers a free online calculator that can solve Algebra 1 problems and provide step-by-step solutions.

Textbooks and Workbooks

• Algebra 1 by Glencoe/McGraw-Hill: A widely used textbook that provides a comprehensive coverage of Algebra 1 concepts.
• Algebra 1 Workbook by Holt McDougal: Offers additional practice problems and exercises to supplement classroom learning.
• Algebra 1: A Complete Introduction by Robert Gerver: A concise and accessible textbook that covers the essential topics of Algebra 1.

Seeking Help

If you encounter difficulties with Algebra 1 concepts, do not hesitate to seek help from your teacher or a tutor.

Teachers can provide personalized guidance, clarify concepts, and offer extra practice problems. Tutors can provide one-on-one support, address specific learning challenges, and help you build confidence in your abilities.

Helpful Answers

What types of equations are covered in this worksheet?

This worksheet covers a variety of equation types, including one-step equations, two-step equations, and equations with variables on both sides.

How do I graph a linear equation?

To graph a linear equation, you need to plot two points and draw a line through them. The slope of the line represents the rate of change, and the y-intercept represents the value of y when x is 0.

What are some real-world applications of linear equations?

Linear equations have numerous real-world applications, such as calculating distance, rate, and time, predicting population growth, and modeling financial situations.